Finding the Area under a Curve using Integration
Look at the plot below of y vs. x. if we want to determine the area of the curve, we can use integration.
NEED SKETCH
First. we sketch a tiny differential element, dA to represent the differential area. The area of dA is y times dx (base time height). So:
![]()
This is the general equation for the area under a curve.
If we want to determine the area between two points under the curve, say a and b, as in the sketch below, we use a and b as the limits:
NEED SKETCH
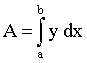
Example: Determine the area under the curve y = x2/2 between 1 and 4.
Sketch the differential area, dA. (Note: this may seem like a trivial step, but it is helping to prepare you for future problems were it is NOT trivial.) THen write the equation for area, A.





