Hyperbola

In a hyperbola, the difference between the distances from two fixed points is constant. These fixed points are the foci of the hyperbola. These points are located at (c,0) and (-c,0).

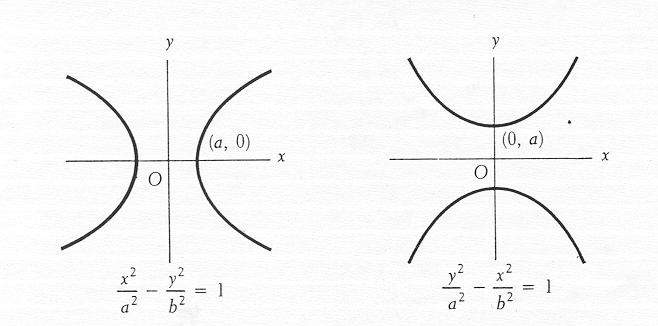
The standard form is: x²/a² – y²/b² = 1

Also: a² + b² = c²

Note that this looks just like the ellipse equation except for the minus sign.

To sketch a hyperbola, you should first sketch its asymptotes. The asymptotes are seen below.

$$$$

EXAMPLE: Sketch x² – 4y² – 16 = 0

Solution: First rearrange this to look like the standard form. How?

Add 16 to both sides. Then divide by 16. You get:

x²/16 – y²/4 = 1

So a = 4 and b = 2. Now connect the points (4,2), (-4,-2) and (4,-2), (-4,2). These two lines are the asymptotes.

Calculate c: c² = a² + b² = 4² + 2²

c = 4.472

So the foci are (4.472, 0) and (-4.472, 0).

Now we can sketch the hyperbola.

$$$$

Click here for practice problems.