The objective of this line of research is to find efficient algorithms for packing an irregularly shaped part with irregular or regular shapes. This falls under geometric packing, a class of mathematical optimization problems with the goal of finding a combination of small objects (bins) that fit a large target (container). Geometric packing in 3D is an understudied field with significant implications in transforming design for sustainable, distributed, and obfuscated compositional manufacturing.
Potential approaches can be partitioning a volume successively into the largest inscribed cubes, few base cubes whose planes cut the outer boundary into covering segments, and a skeleton whose line segments act as principal axes of part body segments (similar to the human body). Examples of the first two approach are shown below.


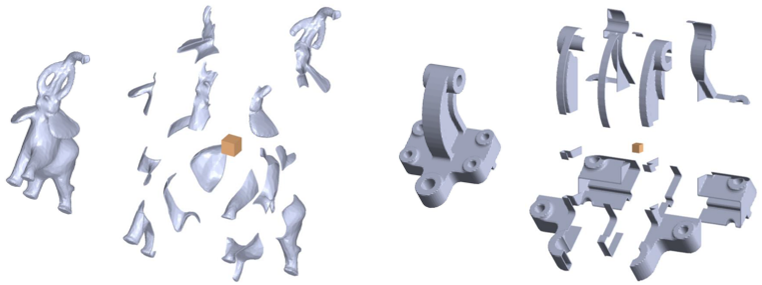
If we succeed in improving and scaling this approach, we help solve a fundamental mathematical problem in synergy with other transformational research. One potential application is in sustainable manufacturing, where we create new functional parts by reusing existing non-functional components or pieces of scrap material. Such an open-ended problem is significantly challenging to solve algorithmically, but humans can creatively tackle it. The examples below show how few participants in an exploratory study created a coat-hanger, book shelf, and a pully from sprues, gates, and miscast parts in a foundry.








