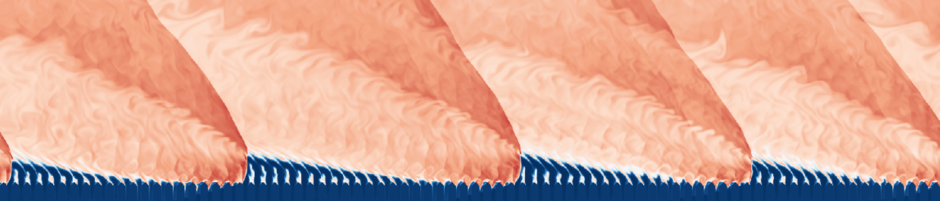
The Rayleigh-Taylor instability is a fundamental problem in fluid mechanics that occurs when a heavy fluid is placed on top of a light fluid. As everyone knows from experience, that is an unstable situation resulting in the inversion of the density stratification. The resulting flow can become very complicated as the two fluids mix through turbulent motions. The turbulence is marked by a wide range of scales of motion, and is thus challenging to describe using numerical simulations. Our approach is to use Implicit Large Eddy Simulations to parse the hierarchy of motions expected in such flows. We use three-dimensional multispecies codes to describe various aspects of this complex flow.
Such flows are important because they have been observed in nuclear fusion, particularly in the Inertial Confinement Fusion (ICF) processes. In ICF, fusion is initiated by laser impingement of a target fuel capsule that leads to implosion of the fuel layers. Here, Rayleigh-Taylor instability can result from irregularities on the fuel-pusher interface. Rayleigh-Taylor instability is also of significance to supernovae explosions. In a supernova detonation (more here), a radially outward blastwave can accelerate the dense stellar material in to the emptiness of space through the Rayleigh-Taylor mechanism. In applications such as ICF or the supernova, the Rayleigh-Taylor instability is driven by a time-varying acceleration, rather than a constant acceleration drive. In our simulations, we have applied Implicit Large Eddy Simulations to understand the effect of such complex acceleration profiles on the development of Rayleigh-Taylor instability, and corresponding mixing outcomes. Data from such simulations are useful in developing and calibrating reduced order models for use in engineering codes.
In the above video, the first clip of RT turbulence shows the time evolution of isosurfaces of density from a 3D simulation in which the acceleration was held constant. As a result, the flow evolves to yield self-similarity, anisotropy in the direction of the applied acceleration, while large structures that dominate the flow at late time are aggregated through coupling of smaller structures constituting an inverse cascade process. Density contours realized at a horizontal midplane clearly highlight the inverse cascade process, as the flow evolves from high-wavenumber initial perturbations to low-wavenumber plumes evident at late times. Naturally, this process is accompanied by significant mixing between the two fluids.
When the flow is driven by the accel-decel-accel profile, the evolution is significantly more complex as evident first in the 3D density isosurface clip, as well as the density contours realized at the horizontal midplane. At the onset of the deceleration, previously growing coherent structures respond by reversing direction. During this stage, the structures are driven by inertia, and collide with each other breaking up in to smaller fragments. This ‘shredding’ (or scrambling) of large scale structures in to smaller fragments is accompanied by a sudden increase in mixing evident by the homogenization of density contours on the horizontal plane. Interestingly, when the destabilizing acceleration is restored, the instability recovers (unscrambling) as the late-stage flow-features resemble the flow field from the constant acceleration simulation. This includes a restoration of anisotropy, self-similarity and the inverse cascade that drives the large-scale development.
Related Publications:
- Y. Zhou, R.J.R. Williams, P. Ramaprabhu et al., “Rayleigh-Taylor and Richtmyer-Meshkov instabilities: A journey through scales”, Physica D, 423, 132838 (2021). https://doi.org/10.1016/j.physd.2020.132838
- G. Dimonte, P. Ramaprabhu & M. Andrews, “Rayleigh-Taylor instability with complex acceleration history”, Phys. Rev. E., 76, 046313, (2007). https://doi.org/10.1103/PhysRevE.76.046313
- P. Ramaprabhu, V. Karkhanis & A.G.W. Lawrie, “The Rayleigh-Taylor instability driven by an accel-decel-accel profile”, Phys. Fluids, 25, 115104 (2013). https://doi.org/10.1063/1.4829765